
Air at 1.013 bar and 25 C with 0.005 specific humidity enters to a compressor with a pressure ratio of 10/1. The isentropic efficiency of the compressor is 0.82. Calculate the temperature, specific enthalpy, and specific entropy of air at the outlet. If the air intake is 5 kg/s, what is the compressor work on the fluid?
| Inputs |
Units |
|
|
Inlet Pressure |
1,013 |
bar |
|
Inlet Temperature |
25,000 |
C |
|
Specific Humidity (moisture content) |
0,005 |
. |
|
Isentropic Efficiency |
0,820 |
. |
|
Pressure Ratio |
10,000 |
. |
|
Mass Flow |
5,000 |
Kg/s |
Equations:
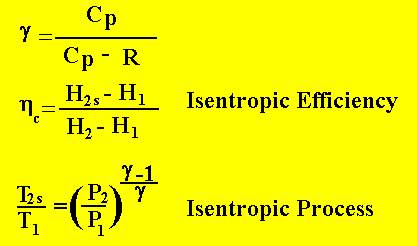
| Outputs | ||
|
Inlet Enthalpy |
37,759 |
kJ/kg |
|
Inlet Entropy |
0,088 |
kJ/(kg.K) |
|
Inlet Specific Heat Capacity |
1,009 |
kJ/(kg.K) |
|
Gas Constant |
0,288 |
kJ/(kg.K) |
|
Gamma |
1,399 |
. |
|
Kelvin |
273,150 |
C |
| . | . | . |
|
Outlet Pressure |
10,130 |
bar |
|
Ln(P2/P1) |
2,303 |
. |
|
(1-1/Gamma)*'Ln(P2/P1) |
0,657 |
. |
|
T2s/T1=.... |
1,929 |
. |
|
T2s |
575,142 |
degree K |
|
T2s |
301,992 |
C |
|
H2s |
321,712 |
kJ/kg |
|
Outlet Enthalpy |
384,043 |
kJ/kg |
|
Outlet Temperature |
360,970 |
C |
|
Outlet Entropy |
0,197 |
kJ/kg |
| . | . | . |
|
Compressor Work on Fluid |
1731,421 |
KJ/s |